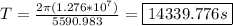Hello!
Recall the period of an orbit is how long it takes the satellite to make a complete orbit around the earth. Essentially, this is the same as 'time' in the distance = speed * time equation. For an orbit, we can define these quantities:
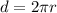 ← The circumference of the orbit
← The circumference of the orbit
speed = orbital speed, we will solve for this later
time = period
Therefore:
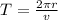
Where 'r' is the orbital radius of the satellite.
First, let's solve for 'v' assuming a uniform orbit using the equation:

G = Gravitational Constant (6.67 × 10⁻¹¹ Nm²/kg²)
m = mass of the earth (5.98 × 10²⁴ kg)
r = radius of orbit (1.276 × 10⁷ m)
Plug in the givens:
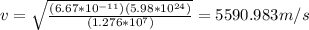
Now, we can solve for the period: