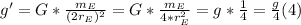Question

Suppose one night the radius of the earth doubled but its mass stayed the same. What would be an approximate new value for the free-fall acceleration at the surface of the earth?

Answer 1

g' = g/4

Step-by-step explanation:

• The value of the free-fall acceleration at the surface of the earth, can be obtained applying Newton's 2nd law, assuming that the only force acting on an object at the surface of the earth, is the one produced by the mass of the Earth, i.e. gravity.
• This force can be expressed according the Newton's Universal Law of Gravitation , as follows:

`F_(g) = G*(m_(x) *m_(E) )/(r_(E)^(2) ) (1)`

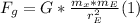
• From Newton's 2nd Law, we have:
• 
  `F = m* a (2)`
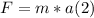
• Since the left sides in (1) and (2) are equal each other, both right sides must be equal each other also.
• Simplifying the mass m, we can write the acceleration a in (2) as the acceleration due to gravity, g, as follows:

`g = G*(m_(E) )/(r_(E)^(2) ) (3)`

• Since G is an universal constant, and the mass mE remains constant, if we double the radius of the Earth, the new value for the acceleration due to gravity (let's call it g'), is as follows:

`g' = G*(m_(E) )/((2r_(E))^(2) ) =G*(m_(E) )/(4*r_(E)^(2) ) = g*(1)/(4) =(g)/(4) (4)`