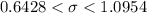Answer:
The 95% confidence interval is
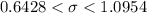
Explanation:
From the question we are told that
The sample size is n = 29
The sample standard deviation is
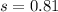
Generally the 95% confidence interval to estimate the true standard deviation is mathematically represented as
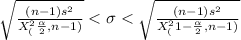
Generally from the chi -distribution table the critical value of
 at a degree of freedom of
at a degree of freedom of
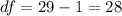 is
is
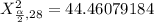
Generally from the chi -distribution table the critical value of
 at a degree of freedom of
at a degree of freedom of
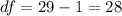 is
is
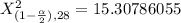
So
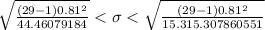
=>