Question

The half-life of cesium-137 is 30 years. Suppose we have a 20 mg sample.

a. Find the mass (in mg) that remains after t years.
b. How much of the sample (in mg) remains after 20 years?
c. After how long will only 1 mg remain?

Answer 1

(a) A = (20mg)/(2^(t/30))

(b) 12.6mg

(c) 129.6years

Explanation:

To calculate the amount remaining after a number of half-lives, n, we can make use of:

`A = (B)/(2^n)`

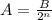
Where A = amount remaining

B = initial amount

`n = (t)/(t_(0.5))`

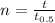
(a) A = (20mg)/(2^(t/30))

(b) Mass after 20years

A = (20mg)/(2^(20/30)) ≈ 12.6mg

(c) After how long will only 1mg remain:

1mg = (20mg)/(2^(t/30))

`20mg = {2^{(t)/(30)}`

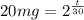
Taking log of both sides we have:

Log(20) = (t/30)log(2)

t/30 = (log(20))/(log(2)) ≈ 4.3

t/30 = 4.3

t = 30 x 4.3 ≈ 129.6years.