Answer:

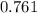
Step-by-step explanation:
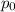 = Stagnation pressure = 52.2 kPa
= Stagnation pressure = 52.2 kPa
 = Atmospheric pressure at 8000 m = 35.581 kPa (from chart)
= Atmospheric pressure at 8000 m = 35.581 kPa (from chart)
M = Mach number
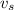 = Velocity of sound at 8000 m = 308 m/s
= Velocity of sound at 8000 m = 308 m/s
v = Velocity of airplane
We have the following equation
![(p_0)/(p)=(1+0.2M^2)^(3.5)\\\Rightarrow M=\sqrt{(1)/(0.2)[((p_0)/(p))^{(1)/(3.5)}-1]}\\\Rightarrow M=\sqrt{(1)/(0.2)[((52.2)/(35.581))^{(1)/(3.5)}-1]}\\\Rightarrow M=0.761](https://img.qammunity.org/2021/formulas/engineering/college/ah1l5pl1q40dbcv1igyuteervi9ttkihoc.png)
Mach number is given by
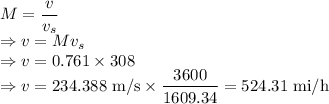
The velocity of the airplance is
 and has a mach number of
and has a mach number of
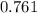 .
.