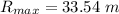Answer:
The value is
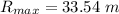
Step-by-step explanation:
From the question we are told that
The total time of flight is

Generally from kinematic equation
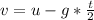
So v is the velocity at maximum height and the value is v = 0 m/s
So
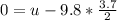
=>
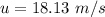
Here u is the initial velocity of the dart as it leaves that gun
Gnerally the horizontal range of the dart is mathematically represented as
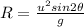
For maximum horizontal range the value of
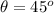
So
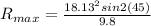
=>