Question

What are all values of x for which the series shown converges? ​

Answer 1

One convergence criteria that is useful here is that, if aₙ is the n-th term of this sequence, then we must have:

Iaₙ₊₁I < IaₙI

This means that the absolute value of the terms must decrease as n increases.

Then we must have:

`((x -2)^n)/(n*3^n) > ((x -2 )^(n+1))/((n + 1)*3^(n+1))`

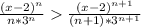
We can write this as:

`((x -2)^n)/(n*3^n) > ((x -2 )^(n+1))/((n + 1)*3^(n+1)) = ((x -2)^n)/((n + 1)*3^n) * ((x - 2))/(3)`

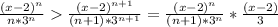
If we assume that n is a really big number, then:

n + 1 ≈ 1

And we can write:

`((x -2)^n)/(n*3^n) > ((x -2)^n)/((n)*3^n) * ((x - 2))/(3)`

Then we have the inequality

`1 > (x - 2)/3`

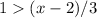
And remember that this must be in absolute value, then we will have that:

-1 < (x - 2)/3 < 1

-3 < x - 2 < 3

-3 + 2 < x < 3 + 2

-1 < x < 5

The first option looks like this, but it uses the symbols ≤≥, so it is not the same as this, then the correct option will be the second.