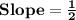Question

Answer the following questions using what you've learned from this unit. Write your responses

in the space provided.
1. Use the slope formula to answer Parts I, II, and III.
Part I: Find the slope of the line that passes through the following points. (6 points; 2 points
each)
A (10,4) and B (-2 , -5) C (-7 ,1) and D (7, 8)

Answer 1

Part a) The slope of line that passes through the points A (10,4) and B (-2 , -5) is
`\mathbf{Slope=(3)/(4) }`

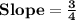
Part b) The slope of line that passes through the points C (-7 ,1) and D (7, 8) is
`\mathbf{Slope=(1)/(2) }`

Explanation:

Part I: Find the slope of the line that passes through the following points.

Part a)

A (10,4) and B (-2 , -5)

We need to find slope of the line that passes through the given points.

The formula used is:
`Slope=(y_2-y_1)/(x_2-x_1)`

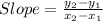
We can take any two points and the slope will be same.

I am taking points A (10,4) and B (-2 , -5) and finding slope

We have
`x_1=10, y_1=4, x_2=-2,y_2=-5`

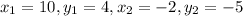
Putting values and finding slope

`Slope=(y_2-y_1)/(x_2-x_1)\\Slope=(-5-4)/(-2-10) \\Slope=(-9)/(-12)\\ Slope=(3)/(4)`

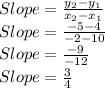
So, the slope of line that passes through the points A (10,4) and B (-2 , -5) is
`\mathbf{Slope=(3)/(4) }`

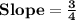
Part a)

C (-7 ,1) and D (7, 8)

We need to find slope of the line that passes through the given points.

The formula used is:
`Slope=(y_2-y_1)/(x_2-x_1)`

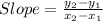
We can take any two points and the slope will be same.

I am taking points C (-7 ,1) and D (7, 8) and finding slope

We have
`x_1=-7, y_1=1, x_2=7,y_2=8`

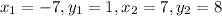
Putting values and finding slope

`Slope=(y_2-y_1)/(x_2-x_1)\\Slope=(8-1)/(7-(-7)) \\Slope=(7)/(14)\\ Slope=(1)/(2)`

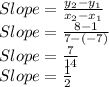
So, the slope of line that passes through the points C (-7 ,1) and D (7, 8) is
`\mathbf{Slope=(1)/(2) }`