Question

Two skaters, a man and a woman, are standing on ice. Neglect any friction between the skate blades and the ice. The mass of the man is 89 kg, and the mass of the woman is 59 kg. The woman pushes on the man with a force of 46 N due east. Determine the acceleration (magnitude and direction) of (a) the man and (b) the woman

Answer 1

Acceleration of this man: approximately
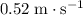`0.52\; {\rm m\cdot s^(-1)}` due east.

Accelerate of this woman: approximately
`0.78\; {\rm m \cdot s^(-1)}` due west.

Step-by-step explanation:

The question implies that the net force on the man is
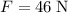`F = 46\; {\rm N}` due east. The mass of the man is
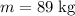`m = 89 \; {\rm kg}`. The acceleration of the man would be:

`\begin{aligned} a &= (F)/(m) \\ &= \frac{46\; {\rm N}}{89\; {\rm kg}} && \genfrac{}{}{0}{}{(\text{due east})}{} \\ &\approx 0.52\; {\rm m\cdot s^(-2)} && (\text{due east})\end{aligned}`.

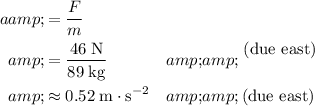In other words, the acceleration of this man would be approximately
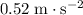`0.52\; {\rm m \cdot s^(-2)}` due east (same direction as the net force.)

By Newton's Laws of Motion, for every force there is a reaction force that is equal in magnitude but opposite in direction.

The woman in this question is applying a due east
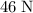`46\; {\rm N}` force on the man. Thus, this woman would experience a reaction force of the same magnitude (
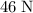`46\; {\rm N}\!`) and opposite direction (due west) from the man. Under assumptions of the question, the net force on the woman would be
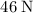`\! 46\; {\rm N}` due west.

The acceleration of this
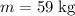`m = 59\; {\rm kg}` woman would be:

`\begin{aligned} a &= (F)/(m) \\ &= \frac{46\; {\rm N}}{59\; {\rm kg}} && \genfrac{}{}{0}{}{(\text{due west})}{} \\ &\approx 0.78\; {\rm m\cdot s^(-2)} && (\text{due west})\end{aligned}`.