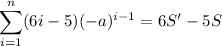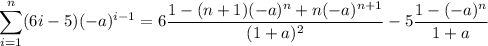Question

Sum to n terms of each of following series. (a) 1 - 7a + 13a ^ 2 - 19a ^ 3+...​

Answer 1

Notice that the difference in the absolute values of consecutive coefficients is constant:

|-7| - 1 = 6

13 - |-7| = 6

|-19| - 13 = 6

and so on. This means the coefficients in the given series

`\displaystyle \sum_(i=1)^\infty c_i a^(i-1) = \sum_(i=1)^\infty |c_i| (-a)^(i-1) = 1 - 7a + 13a^2 - 19a^3 + \cdots`

occur in arithmetic progression; in particular, we have first value
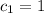`c_1 = 1` and for
`n>1`,
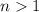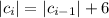`|c_i|=|c_(i-1)|+6`. Solving this recurrence, we end up with

`|c_i| = |c_1| + 6(i-1) \implies |c_i| = 6i - 5`

So, the sum to
`n` terms of this series is

`\displaystyle \sum_(i=1)^n (6i-5) (-a)^(i-1) = 6 \underbrace{\sum_(i=1)^n i (-a)^(i-1)}_(S') - 5 \underbrace{\sum_(i=1)^n (-a)^(i-1)}_S`

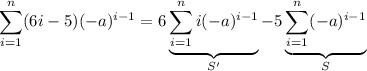
The second sum
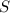`S` is a standard geometric series, which is easy to compute:

`S = 1 - a + a^2 - a^3 + \cdots + (-a)^(n-1)`

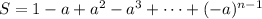
Multiply both sides by
`-a` :

`-aS = -a + a^2 - a^3 + a^4 - \cdots + (-a)^n`

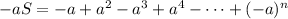
Subtract this from
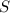`S` to eliminate the intermediate terms to end up with

`S - (-aS) = 1 - (-a)^n \implies (1-(-a)) S = 1 - (-a)^n \implies S = (1 - (-a)^n)/(1 + a)`

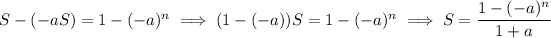
The first sum
`S'` can be handled with simple algebraic manipulation.

`S' = \displaystyle \sum_(i=1)^n i (-a)^(i-1)`

`\displaystyle S' = \sum_(i=0)^(n-1) (i+1) (-a)^i`

`\displaystyle S' = \sum_(i=0)^(n-1) i (-a)^i + \sum_(i=0)^(n-1) (-a)^i`

`\displaystyle S' = \sum_(i=1)^(n-1) i (-a)^i + \sum_(i=1)^n (-a)^(i-1)`

`\displaystyle S' = \sum_(i=1)^n i (-a)^i - n (-a)^n + S`

`\displaystyle S' = -a \sum_(i=1)^n i (-a)^(i-1) - n (-a)^n + S`

`\displaystyle S' = -a S' - n (-a)^n + (1 - (-a)^n)/(1 + a)`

`\displaystyle (1 + a) S' = (1 - (-a)^n - n (1 + a) (-a)^n)/(1 + a)`

`\displaystyle S' = (1 - (n+1)(-a)^n + n (-a)^(n+1))/((1+a)^2)`

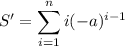
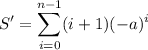
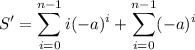
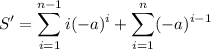
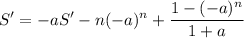
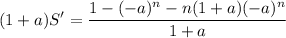
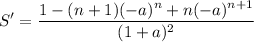
Putting everything together, we have

`\displaystyle \sum_(i=1)^n (6i-5) (-a)^(i-1) = 6 S' - 5 S`

`\displaystyle \sum_(i=1)^n (6i-5) (-a)^(i-1) = 6 (1 - (n+1)(-a)^n + n (-a)^(n+1))/((1+a)^2) - 5 (1 - (-a)^n)/(1 + a)`

`\displaystyle \sum_(i=1)^n (6i-5) (-a)^(i-1) =\boxed{(1 - 5a - (6n+1) (-a)^n + (6n-5) (-a)^(n+1))/((1+a)^2)}`