Question

For constant volume processes the heat capacity of gas A is greater than the heat capacity of gas B. We conclude that when they both absorb the same energy as heat at constant volume:

A. the temperature of A increases more than the temperature of B
B. the temperature of B increases more than the temperature of A
C. the internal energy of A increases more than the internal energy of B
D. the internal energy of B increases more than the internal energy of A
E. A does more positive work than B

Answer 1

B). the temperature of B increases more than the temperature of A

Step-by-step explanation:

For constant volume process, relation between molar heat capacity and temperature is given by,

`$\Delta Q = mC_v \Delta T$`

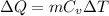
where, ΔQ is the amount of heat required

m is the mass of the gas

`$C_v$` is heat capacity at constant volume

ΔT is the change in temperature.

For a constant energy ΔQ,

`$C_v=(1)/(\Delta T)$`

Given,
`$(C_v)_A>(C_v)_B$`

∴
`$(\Delta T)_A < (\Delta T)_B $`

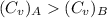
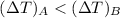
Thus the temperature of gas B increases more than the temperature of gas A.