Given:

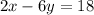
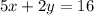
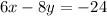
To find:
The slope intercept form of each equation.
Solution:
We know that, slope intercept form of a line is

where, m is slope and b is y-intercept.
The first equation is

Subtract 6x from both sides.
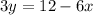
Divide both sides by 3.
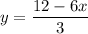
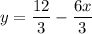
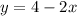
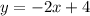
Therefore, the slope intercept form of first equation is
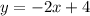 .
.
Similarly,
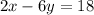
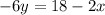

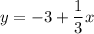
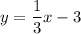
Therefore, the slope intercept form of second equation is
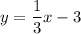 .
.
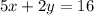

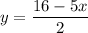


Therefore, the slope intercept form of third equation is
 .
.
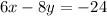
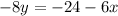
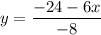

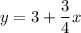
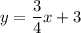
Therefore, the slope intercept form of fourth equation is
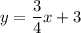 .
.