Question

Martha is late for class, so she races her 75 kg body up a 4.0 meter flight of stairs in only 2.3 seconds. What is Martha’s power?

Answer 1

Martha's power can be calculated by finding the work done and then dividing it by the time taken. The work done is equal to the change in potential energy, which is calculated using the formula: Work = mgh. Given that Martha's mass is 75 kg, the height of the flight of stairs is 4.0 m, and the time taken is 2.3 seconds, Martha's power is 1278 W.

Step-by-step explanation:

Power is the rate at which work is done or energy is transferred, and it is calculated using the formula: Power = Work / Time. In this case, Martha's power can be calculated by first finding the work done and then dividing it by the time taken. The work done is equal to the change in potential energy, which can be calculated using the formula: Work = mgh, where m is the mass, g is the acceleration due to gravity, and h is the height.

Given that Martha's mass is 75 kg, the height of the flight of stairs is 4.0 m, and the time taken is 2.3 seconds, we can calculate the work done: Work = (75 kg)(9.8 m/s^2)(4.0 m) = 2940 J.

Finally, we can calculate Martha's power: Power = Work / Time = 2940 J / 2.3 s = 1278 W.

Answer 2

`1.3\cdot 10^3\text{ J}`

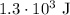
Step-by-step explanation:

Power is defined by work over time. In physics, work can be defined as the energy transferred over from an applied force over some distance. As a formula:

`W=Fd`, where F = force applied and d = distance that force is applied over.

*It is worth noting that F must be parallel to the distance travelled. If it is perpendicular, no work is done, and if it is at an angle, find the parallel component and use that for F.

In this case, the force applied must counter the force of gravity on Martha, which is given by
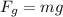`F_g=mg`, where m = Martha's mass and g = gravitational constant 9.8 m/s/s. Therefore,
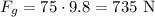`F_g=75\cdot 9.8=735\text{ N}`. Since she raises her body 4.0 meters, the work done must be
`W=Fd=735\cdot 4=2940\text{ J}`.

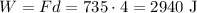Since power is equal to work over time and t = 2.3 seconds, we have:

`\displaystyle P=(W)/(t)=(2940)/(2.3)=\boxed{1278\text{ J}}\approx \boxed{1.3\cdot 10^3\text{ J}}` (to two significant figures)