Question

A rotating shaft is subjected to a steady torsional stress of 13 ksi and an alternating bending stress of 22 ksi.

(A) Find the yielding factor of safety using the distortion energy theory.
(B) Find the fatigue factor of safety using the Goodman criterion. A shaft has the properties of Se= 35 ksi, Sy = 60 ksi, and Sut = 85 ksi.

Answer 1

A) б1 = 28 ksi and б2 = -6.02 ksi

B) 1.25

Step-by-step explanation:

Given data :

Torsional stress = 13 ksi

Alternating bending stress = 22ksi

A) determine yielding factor of safety according to the distortion energy theory

б1,2 =
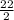`(22)/(2)` ± √(22/2)² + 13²

= 11 ± 17

therefore б1 = 28 ksi hence б2 = -6.02 ksi

B) determine the fatigue factor of safety

with properties ; Se = 35ksi, Sy = 60 ksi, Sut = 85 ksi

( б1 - б2 )² + ( б2 - б3 )² + ( б3 - б1 )² ≤ 2 ( Sy / FOS ) ²

( 28 + 6.02 ) ² + ( 6.02 - 0 )² + ( 0 - 28 )² ≤ 2 ( 60 / FOS ) ²

solving for FOS = 1.9

Next we can determine FOS with the use of Goodman criterion

бm / Sut + бa / Se = 1 / FOS

= 0 / 85 + 28/35 = 1 / FOS

making FOS the subject of the equation ; hence FOS = 1.25