__________________________________________________________
__________________________________________________________

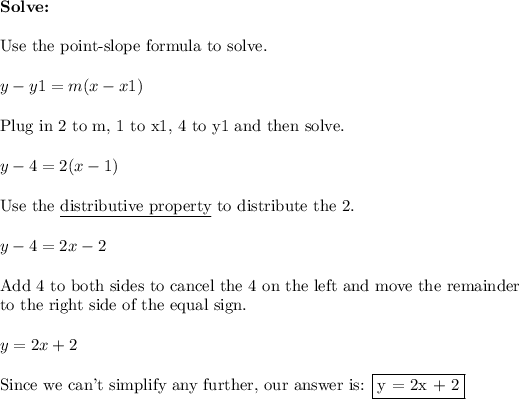
__________________________________________________________
__________________________________________________________
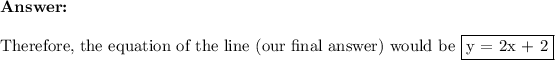
__________________________________________________________