Question

A store has two different sizes of ice cream the smaller container cost 3.87 for 48 ounces the larger container cost 6.42 which ice cream cost less per ounce

Answer 1

Consider the complete question is "A store has two different sizes of ice cream on sale this week. The smaller container costs $3.87 for 48 ounces. The larger container costs $6.42 for 128 ounces. which ice cream costs less per ounce?"

Given:

The smaller container costs $3.87 for 48 ounces.

The larger container costs $6.42 for 128 ounces.

To find:

Which ice cream costs less per ounce?

Solution:

We know that

`\text{Cost per ounce}=\frac{\text{Total cost}}{\text{Number of ounces}}`

Using this formula, we get

Per ounce cost of small container
`=(3.87)/(48)`

`=0.080625`

`\approx 0.08`

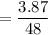
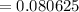
Per ounce cost of larger container
`=(6.42)/(128)`

`=0.05015625`

`\approx 0.05`

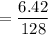
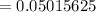
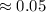
Since, 0.08 > 0.05 therefore, the per ounce cost of larger container is less.