Answer:
There is no point where x = 3.5
Explanation:
Given the inequality
5x – 3 > 14.5
We are to find the range of all values of x
Add 3 to both sides of the equation;
5x - 3 + 3 > 14.5+ 3
5x > 17.5
x > 17.5/5
x > 3.5
Also for the inequality;
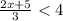
Cross multiply;
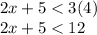
Subtract from both sides;
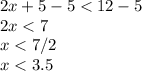
The solution to both inequalities are x > 3.5 and x < 3.5. Since there is no point where x = 3.5, hence the solution will have solution on all real numbers except at x = 3.5