Question

A Super Happy Fun Ball is dropped from a height of 4 feet and rebounds 4 5 of the distance from which it fell. How many times will it bounce before its rebound is less than 1 foot

Answer 1

More than 6.21 times

Explanation:

The common ratio
`r=(4)/(5)`

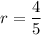
Distance of first bounce

`a_1=4* (4)/(5)=(16)/(5)`

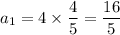
Second bounce

`a_2=ar^(n-1)=(16)/(5)((4)/(5))^(2-1)\\\Rightarrow a_2=(16)/(5)((4)/(5))`

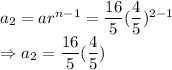
n bounce

`a_n=ar^(n-1)\\\Rightarrow a_n=(16)/(5)((4)/(5))^(n-1)`

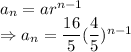
Now the nth bounce will be less than 1 feet

`(16)/(5)((4)/(5))^(n-1)<1\\\Rightarrow ((4)/(5))^(n-1)<(5)/(16)`

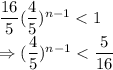
Applying logarithms on both sides we get

`(n-1)\ln (4)/(5)<\ln(5)/(16)\\\Rightarrow n>(\ln(5)/(16))/(\ln (4)/(5))+1`

The inequality changes as both
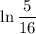`\ln(5)/(16)` and
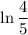`\ln (4)/(5)` are negative numbers.

So,

`n>6.21`

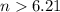
Hence, the number of bounces is more than 6.21 times.