Question

Solve for x in the diagram below.

Answer 1

x = 20

Explanation:

Key points:

• understanding diagram notation
• understanding the Angle Addition Postulate

Diagram notation

In the diagram, there are three angles, each with an expression representing the measure of their angle (in degrees). Angles are adjacent (next to each other with the same vertex) such that collectively they form one large angle.

The small square in the lower left corner means that the large angle is a right angle. All right angles are 90°.

Note: The expressions for each angle all have a degree marking, so each angle is measured in degrees. The unknown value x, is a number without any units, the expression is simplified, and then the units "degrees" are applied. So in the end, the answer for "x" will not have units; "x" will just be a number.

Angle Addition Postulate

The Angle Addition Postulate states that the sum of the measures of two adjacent angles is equal to the measure of the large angle formed by those adjacent angles.

`(\text{measure of first angle})+(\text{measure of second angle})=(\text{measure of the combined angle})`

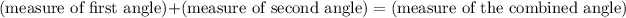
Solving the given problem

By applying the Angle Addition Postulate a couple times, the sum of the measures of all three angles in the diagram is equal to the measure of the large angle formed by those three angles.

`(\text{measure of first angle})+(\text{measure of second angle})+(\text{measure of third angle})=(\text{measure of the large right angle})`

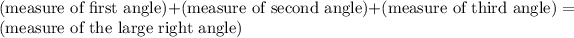
or

`(x)+(2x)+(x+10)=(90)`

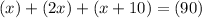
From here, to solve for x, we'll need to combine like terms, isolate x, and simplify.

Start with the Associative Property of Addition to combine like terms:

`(x+2x+x)+10=90\\4x+10=90`

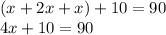
To isolate x, subtract 10 from both sides and simplify...

`(4x+10)-10=(90)-10\\4x=80`

... then divide by 4 on both sides and simplify.

`\frac {4x}{4}=\frac {80}{4}\\x=20`

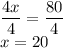
So, x=20

Answer 2

x = 20°

Explanation:

x° + 2x°+ (x+10)° = 90°

4x° + 10° = 90°

4x° = (90 -10)°

x° = (80/4)°

x = 20°

Hope this helps