Question

For which value of x is the expression y

=
a. 1
b. -1
C. 5
d. -5
3x-3
x-5
- undefined?

Answer 1

1

Explanation:

when we put 1 in the equation the nominator becomes zero and it becomes undefined

Answer 2

Hii!

___________________________________________________________

`\stackrel\star\rightsquigarrow\circ\boldsymbol{\underbrace{Answer:}}}\circ\leftharpoonup`

The value of x should be 5! ^^

`\stackrel\star{\rightsquigarrow\circ\boldsymbol{\underbrace{Explanation:}}}\circ\leftharpoonup`

`\boxed{\\\begin{minipage}{7cm} For\,an\,expression \\ \boldsymbol{to\,be\,und efined,} \\ \,its\,denominator\,should\,be\,zero \end{minipage}}}`

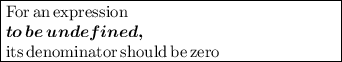
Remember this! ^^

Now let's consider this:

Which value of x will make the denominator equal to 0?

Is it 1? If we stick 1 in, we will not obtain 0.

`\twoheadrightarrow\sf y=\cfrac{3\cdot1-3}{1-5}`

`\bullet` Simplify this

`\twoheadrightarrow\sf y=\cfrac{3-3}{-4}`

---

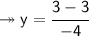
We will obtain 0 in the numerator, but not in the denominator; if the numerator is 0, then the fraction, or fractional expression, is 0.

Let's try 5. 5-5 is equal to 0, so this sounds promising! ^^

`\twoheadrightarrow\sf y=\cfrac{3\cdot5-3}{5-5}`

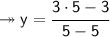
`\bullet` Look what we obtain when we simplify

Don't we obtain 15-3/0?

Like I said above, if an expression has 0 as its denominator, it's undefined.

--

Hope that this helped! Best wishes.

`\textsl{Reach far. Aim high. Dream big.}`

--