Answer:
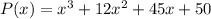
Explanation:
Roots of a polynomial
If we know the roots of a polynomial, say x1,x2,x3,...,xn, we can construct the polynomial using the formula
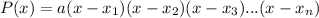
Where a is an arbitrary constant.
We are given the following roots:
-5 multiplicity 2 (-5 twice)
-2 multiplicity 1
Thus, the polynomial is:
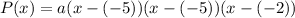
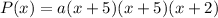
We are not given any clue about the value of a, so we choose a=1. Multiplying:
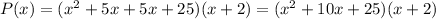
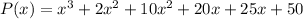
Simplifying:
