Question

Identify the type of function represented by f(x)= 3/8(4)^x

Answer 1

It is an exponential function

Explanation:

We have the following function:

f (x) = 3/8 (4) ^ x

The function is of the form:

f (x) = A (b) ^ x

Where,

A: initial amount

b: growth factor (b> 1)

x: time variable.

Answer:

f (x) = 3/8 (4) ^ x

It is an exponential function of the form:

f (x) = A (b) ^ x

Answer 2

The function represented by f(x) =
`(3)/(8)`
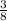
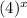`(4)^(x)` is an exponential function.

An exponential function is characterized by having a constant base raised to the variable power. In this case, the base is 4, and x is the exponent.

The coefficient
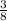`(3)/(8)` scales the function's output.

The general form of an exponential function is f(x)=a⋅
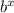`b^(x)`, where

a is a constant multiplier, and

b is the base

So, the function f(x) =
`(3)/(8)`
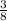
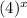`(4)^(x)` is a specific instance of an exponential function, where the base is 4, and the coefficient
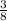`(3)/(8)` scales the exponential growth. Understanding these characteristics helps analyze the behavior of the function as x varies.