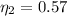Answer:
The value is
Step-by-step explanation:
From the question we are told that
The temperature of the heat source is
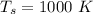
The amount of power transferred is
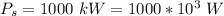
The work produced is
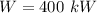
The temperature of the environment
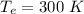
Gnerally the Carnot efficiency of the system is mathematically represented as
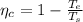
=>
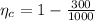
=>
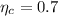
Generally the first law efficiency of the system is mathematically represented as
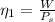
=>
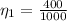
=>
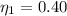
Generally the second law efficiency of the system is mathematically represented as
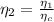
=>
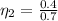
=>