Question

In triangle ABC, the measure of angle B is 18° more than twice the measure of angle A. The measure of angle C is 42° more than that of angle A. Find the angle measures. A: 30°, 78°, 42° B: 30°, 78°, 72° C: 33°, 84°, 63° D: 26°, 70°, 84°

Answer 1

B: 30°, 78°, 72

Explanation:

Given a
`\triangle ABC`.

Let
`\angle A = x^\circ`

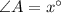
As per question statement,
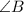`\angle B` is 18° more than twice of
`\angle A`.

i.e

`\angle B =(2x+18)^\circ`

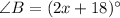
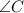`\angle C` is 42° more than that of
`\angle A`

`\angle C = (x+42)^\circ`

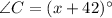
To find:

The angle measures of the triangle = ?

Solution:

We can use the angle sum property of a triangle here to find the three angles of the triangle.

As per angle sum property, the sum of all the three internal angles is equal to
`180^\circ`.

`\angle A + \angle B + \angle C= 180^\circ\\\Rightarrow x+2x+18+x+42=180^\circ\\\Rightarrow 4x+60=180\\\Rightarrow 4x=120\\\Rightarrow x= 30^\circ`

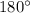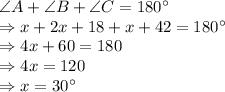
i.e.
`\angle A = 30^\circ`

`\angle B = 2* 30 + 18 = 78^\circ`

`\angle C = 30+42 = 72^\circ`

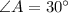
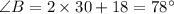
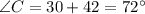
Therefore, the correct answer is:

B: 30°, 78°, 72