Given:
Two points that are close to the line of best fit are (2,70) and (4.5, 90).
To find:
Th equation of best fit line using the given two points.
Solution:
We know that, if a line passes through the two points, then the equation of line is

Two points (2,70) and (4.5, 90) are close to the best fit line.
Consider the two points (2,70) and (4.5, 90). So, the equation of line is
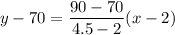

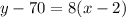
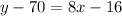
Adding 70 on both sides, we get
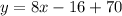
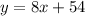
Therefore, the equation of best fit line is
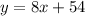 .
.