Given:
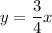

To find:
Whether the above functions are proportional or non-proportional?
Solution:
If y is proportional to x, then
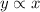
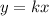
where, k is constant of proportionality.
The proportional relationships are in the form of
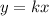 and they passes through (0,0).
and they passes through (0,0).
Putting x=0 and y=0 in the given equations.
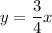
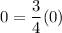
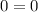
It is a true statement.

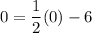
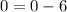
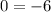
It is a false statement.
Therefore,
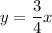 is proportional and
is proportional and
 is non-proportional.
is non-proportional.