Question

write the x and y coordinate (in the second and third column, respectively) of dilation of quadrilateral ABCD with vertices A(1,1), B(2,2), C(4,1) and d(2,-1). Use a scale factor of 2

Answer 1

The coordinates of the dillated vertices are
`A'(x,y) = (2,2)`,
`B'(x,y) = (4,4)`,
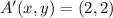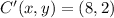`C'(x,y) = (8,2)` and
`D'(x,y) = (4,-2)`.

Explanation:

From Linear Algebra, we define dilation by the following equation:

`P'(x,y) = O(x,y) + k\cdot [P(x,y)-O(x,y)]` (1)

Where:

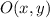`O(x,y)` - Center of dilation, dimensionless.

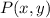`P(x,y)` - Original point, dimensionless.

`k` - Scale factor, dimensionless.

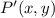`P'(x,y)` - Dilated point, dimensionless.

If we know that
`O(x,y) = (0, 0)`,
`k = 2`,
`A(x,y) = (1,1)`,
`B(x,y) = (2,2)`,
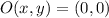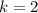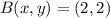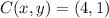`C(x,y) = (4,1)` and
`D(x,y) = (2,-1)`, then the dilated points are, respectively:

Point A

`A'(x,y) = O(x,y) + k\cdot [A(x,y)-O(x,y)]` (2)

`A'(x,y) = (0,0) + 2\cdot [(1,1)-(0,0)]`

`A'(x,y) = (2,2)`

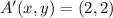
Point B

`B'(x,y) = O(x,y) + k\cdot [B(x,y)-O(x,y)]` (3)

`B'(x,y) = (0,0) + 2\cdot [(2,2)-(0,0)]`

`B'(x,y) = (4,4)`

Point C

`C'(x,y) = O(x,y) + k\cdot [C(x,y)-O(x,y)]`

`C'(x,y) = (0,0) + 2\cdot [(4,1)-(0,0)]`

`C'(x,y) = (8,2)`

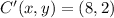
Point D

`D'(x,y) = O(x,y) + k\cdot [D(x,y)-O(x,y)]`

`D'(x,y) = (0,0) + 2\cdot [(2,-1)-(0,0)]`

`D'(x,y) = (4,-2)`

The coordinates of the dillated vertices are
`A'(x,y) = (2,2)`,
`B'(x,y) = (4,4)`,
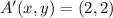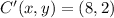`C'(x,y) = (8,2)` and
`D'(x,y) = (4,-2)`.