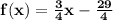Question

13

A local business is calculating the total profit made f(x) after selling
x cupcakes. The business would like to predict their profit by creating an equation for the function.

Write an equation that models the total profit for the business below.
​Drag and drop the numbers into the boxes to create the function's equation. Not all numbers listed will be used.

x | f(x)​​
_____
3 | -5
7 | -2
11 | 1
15 |4
(it won’t allow me to send another pic with the chart so i hope this helps. & the picture i did attach are the things you have to use to create the problem it has to be in the form f(x) = _ x + _

Answer 1

The required equation is:
`\mathbf{f(x)=(3)/(4)x-(29)/(4)}`

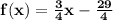
Explanation:

We need to find equation from the table given

x f(x)

3 -5

7 -2

11 1

15 4

We can write equation in the form of
`y=mx+b`

where m is slope and b is y-intercept.

Finding Slope

We can used the slope formula to find slope:
`Slope=(y_2-y_1)/(x_2-x_1)`

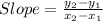
From the table we have:
`x_1=3, y_1=-5, x_2=7, y_2=-2`

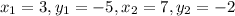
Putting values and finding slope

`Slope=(y_2-y_1)/(x_2-x_1)\\Slope=(-2-(-5))/(7-3) \\Slope=(-2+5)/(7-3)\\Slope=(3)/(4)`

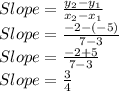
So, we get slope
`m=(3)/(4)`

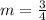
Now, finding y-intercept

Using the slope
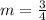`m=(3)/(4)` and point (3,-5) we can find y-intercept

`y=mx+b\\-5=(3)/(4)(3)+b\\-5=(9)/(4)+b\\b=-5-(9)/(4)\\b=(-5*4-9)/(4)\\b=(-20-9)/(4)\\b=(-29)/(4)`

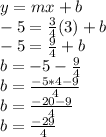
The y-intercept is
`b=(-29)/(4)`

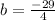
So, the equation having slope
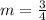`m=(3)/(4)` and y-intercept
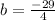`b=(-29)/(4)` will be:

`y=mx+b\\y=(3)/(4)x-(29)/(4)`

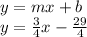
The required equation is:
`\mathbf{f(x)=(3)/(4)x-(29)/(4)}`