Answer:
a) the platform is 110 feet above the ground.
b) It takes 8.69 sec for the rock to hit the ground
Explanation:
This situation is modelled by the following function:
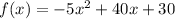
We need to answer the following questions.
a) How high above the ground is the platform?
First we find x using the formula:
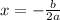
we have b = 40 and a = -5
Putting values and finding x
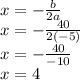
Now putting x=4 in the function to find how high above the ground is the platform
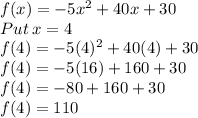
So, the platform is 110 feet above the ground.
b) How long does it take for the rock to hit the ground? Show all work to receive full credit!
We will factorise the given function
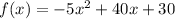 to find the time taken to hit the ground.
to find the time taken to hit the ground.
We can solve the equation using quadratic formula:
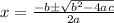
We have a =-5, b=40, c=30
Putting values and finding x
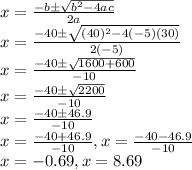
So, we get values of x: x = -0.69 and x=8.69
As time cannot be negative, so we take x=8.69
So, It takes 8.69 sec for the rock to hit the ground