Given that,
The coordinates of A = (-10,-8)
The coordinates of B = (80,37)
To find,
The coordinates of a point that partitions segment AB in a ratio of 4:5.
Solution,
Let the coordinate of required point is (x,y). We can use section formula to find it as follows :
The section formula is :
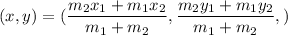
We have,
m₁ =4, m₂ = 5, x₁ = -10, x₂ = 80, y₁ = -8, y₂ = 37
Using all the values,
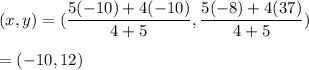
So, the required point in which AB is divided is (-10,12)