Question

An airplane travels at 500.0 km/hr W. It encountered a south bound cross-wind and ends up with a velocity of 625 km/hr SW. What was the velocity of the cross-wind? Be sure to record your answer to the correct precision!

Answer 1

The velocity of the cross-wind was 375 km/hr South

Step-by-step explanation:

The given speed of the airplane, v₀ = 500.0 km/hr W

The direction the airplane is headed = West

The direction of the wind encountered = Southbound wind

The final velocity of the airplane, R = 625 km/hr

The final direction of the airplane = South West SW

Let vₐ represent the velocity of the cross-wind

Therefore, we have the given velocities in vector form as follows;

R = -500.0·i - vₐ·j

Also, we have;

`\left | R \right | = 625 = √(v_0^2 + v_a^2) = √((-500)^2 + (-v_a)^2)`

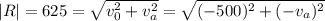
625² = (-500)² + (-vₐ)²

(-vₐ)² = 625² - (-500)² = 140625

-vₐ = 375

vₐ = -375 km/hr North = 375 km/hr South

The velocity of the cross-wind = vₐ = 375 km/hr South