Question

When a falling meteoroid is at a distance above the Earth's surface of 3.40 times the Earth's radius, what is its acceleration due to the Earth's gravitation

Answer 1

g = 0.85 m
`s^(-2)`

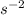
Step-by-step explanation:

g =
`(GM)/(h^(2) )`

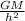
were; g is the acceleration due to Earth's gravity, G is Newton's gravitation constant (6.674 x
`10^(-11)` N
`m^(2)`
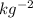`kg^(-2)`), M is the mass of the earth (5.972 x
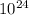`10^(24)` kg), and h is the distance of meteoroid to the earth.

h = 3.40 x R

= 3.40 x 6371 km

h = 21661.4 km

= 21661400 m

Thus,

g =
`(6.674*10^(-11)*5.972*10^(24) )/((21661400)^(2) )`

=
`(3.9857 *10^(14) )/(4.6922*10^(14) )`

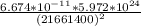
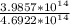
= 0.84944

g = 0.85 m
`s^(-2)`

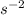
The acceleration due to the Earth's gravitation is 0.85 m
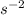`s^(-2)`.