Answer:
The largest advertisement she can afford has dimensions of 5 in x 10/3 in
Explanation:
Assume the dimensions of the advertisement are L and W.
The area of the advertisement is:
A = L.W
The ratio length/width is 3:2, thus the proportion is:
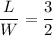
Thus:
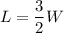
The area is:
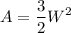
Since the square inch of advertisement space sells for $3, the cost for Anna to purchase it is:
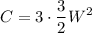
Simplifying:
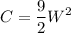
This cost can be a maximum of $50, thus:

Multiplying by 2:
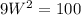
Solving for W:

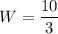
And

L = 5
Thus the largest advertisement she can afford has dimensions of 5 in x 10/3 in