Question

If x = 3 (y + 2) minus 1 what is the value of w in terms of x and y?

w = StartFraction x minus 3 y Over 3 EndFraction
w = StartFraction x minus 3 y + 1 Over 3 EndFraction
w = x minus 3 y + 1
w = StartFraction x + 1 Over 9 y EndFraction

Answer 1

`w=(x-3y+1)/(3)`

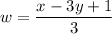
Explanation:

The given expression is :

x = 3 (y + w) -1

We need to find the value of w in terms of x and y.

Solving RHS of the given expression :

x = 3y+3w-1

subtracting both sides 3y.

x-3y = 3y+3w-1 -3y

x-3y = 3w-1

Add 1 both sides

x-3y+1=3w-1+1

3w=x-3y+1

`w=(x-3y+1)/(3)`

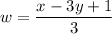
Hence, the correct option is (c) "
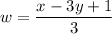`w=(x-3y+1)/(3)` i.e. w = StartFraction x minus 3 y + 1 Over 3 EndFraction.