Given:
The quadratic equation is
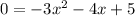
To find:
The simplest radical form of the solution.
Solution:
Quadratic formula:
If a quadratic equation is
 , then
, then
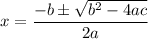
We have,
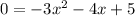
Here, a=-3, b=-4 and c=5. Putting these values in the quadratic formula, we get
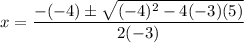
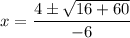
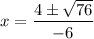
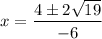
Taking 2 common, we get
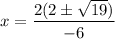
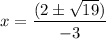
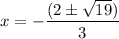
Therefore, the correct option is A.