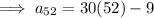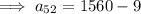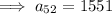Question

Find the common difference, the 52nd term, and the SIMPLIFIED general term formula.

1) 21, 51, 81, 111, ….

I need this ASAP!!!!! PLEASE I’M begging you!!!!

Answer 1

Common difference: 30

General term formula:
`a_n=30n-9`

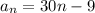
52nd term: 1551

Explanation:

Given sequence:

21, 51, 81, 111

Calculate the difference in terms:

`21 \underset{+30}{\longrightarrow} 51 \underset{+30}{\longrightarrow} 81 \underset{+30}{\longrightarrow} 111`

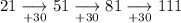
The sequence is increasing by 30 each time, so as there is a common difference, this is an arithmetic sequence with a common difference of 30.

General form of an arithmetic sequence

`a_n=a+(n-1)d`

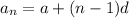
where:

• 
  `a_n` is the nth term
• a is the first term
• d is the common difference between consecutive terms

Given:

• a = 21
• d = 30

Substitute the given values into the formula to find the general term formula:

`\implies a_n=21+(n-1)30`

`\implies a_n=21+30n-30`

`\implies a_n=30n-9`

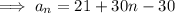
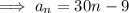
To find the 52nd term, simply substitute n = 52 into the found general term formula:

`\implies a_(52)=30(52)-9`

`\implies a_(52)=1560-9`

`\implies a_(52)=1551`