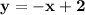Question

Write an equation in

slope-intercept form
to represent the
relationship on the
graph.

Answer 1

The equation in slope-intercept form of required line is:
`\mathbf{y=-x+2}`

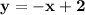
Explanation:

We need to write equation in slope-intercept form to represent the relationship on the graph.

The general equation in slope-intercept form is:
`y=mx+b`

Where m is slope and b is y-intercept

Finding slope using formula:
`Slope=(y_2-y_1)/(x_2-x_1)\\`

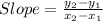
Looking at he graph we have

x_1=0, y_1=2, x_2=1, y_2=1

Putting values in formula and finding slope:

`Slope=(y_2-y_1)/(x_2-x_1)\\\\Slope=(1-2)/(1-0)\\Slope=(-1)/(1)\\Slope=-1`

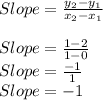
So, we get slope m = -1

Using slope m=-1 and point (0,2) we can find y-intercept

`y=mx+b\\2=-1(0)+b\\b=2`

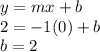
So, y-intercept b = 2

The equation of line having m=-1 and b=2 will be:

`y=mx+b\\y=-1(x)+2\\y=-x+2`

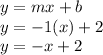
The equation in slope-intercept form of required line is:
`\mathbf{y=-x+2}`