Question

Write an equation in

slope-intercept form to
represent the relationship
shown in the table.

Answer 1

Equation in slope-intercept form is
`\mathbf{y=3x}`

Explanation:

We need to write equation in slope-intercept form to represent the relationship shown in the table.

The general equation of slope-intercept form is:
`y=mx+b`

where m is slope and b is y-intercept.

Finding slope using point (-2,-6) and (0,0)

The formula used is:
`Slope=(y_2-y_1)/(x_2-x_1)`

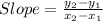
We have
`x_1=-2, y_1=-6, x_2=0, y_2=0`

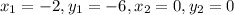
Putting values and finding slope

`Slope=(y_2-y_1)/(x_2-x_1)\\Slope=(0-(-6))/(0-(-2))\\Slope=(6)/(2)\\Slope=3`

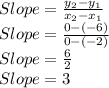
Using slope m= 3 and point (-2,-6) we can find y-intercept

`y=mx+b\\-6=3(-2)+b\\-6=-6+b\\b=-6+6\\b=0`

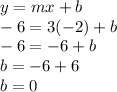
So, we have y-intercept b =0

Equation in slope-intercept form having slope m= 3 and y-intercept b =0 is:

`y=mx+b\\y=3x+0\\y=3x`

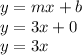
So, Equation in slope-intercept form is
`\mathbf{y=3x}`