Final Answer:
If the
 in the equation
in the equation
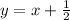 is replaced by -2, the new equation becomes
is replaced by -2, the new equation becomes
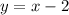 . The statement about the graph that will be true is that the new line will have the same slope as the original line but a different y-intercept.
. The statement about the graph that will be true is that the new line will have the same slope as the original line but a different y-intercept.
Explanation:
When replacing
 with -2 in the equation
with -2 in the equation
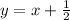 , we get
, we get
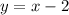 . This transformation changes the y-intercept of the line without affecting its slope. The original equation
. This transformation changes the y-intercept of the line without affecting its slope. The original equation
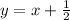 has a y-intercept of
has a y-intercept of
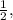 meaning the line crosses the y-axis at the point
meaning the line crosses the y-axis at the point
 . On the other hand, the new equation
. On the other hand, the new equation
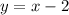 has a y-intercept of -2, so the line crosses the y-axis at the point (0, -2).
has a y-intercept of -2, so the line crosses the y-axis at the point (0, -2).
The slope of both equations is 1, as indicated by the coefficient of x. Therefore, the lines have the same steepness or inclination. The change in the y-intercept, however, results in a vertical shift of the graph. Visually, the new line will be parallel to the original line but will be shifted downward on the coordinate plane.
This shift doesn't alter the slope, emphasizing that the replacement of
 with -2 only affects the position of the line along the y-axis. In summary, the statement about the graph being true means recognizing that the replacement changes the y-intercept while maintaining the original slope.
with -2 only affects the position of the line along the y-axis. In summary, the statement about the graph being true means recognizing that the replacement changes the y-intercept while maintaining the original slope.