Question

|x+2|<4 absolute value

Answer 1

-6 < x < 2

Explanation:

This is the simplest form of absolute value inequality. It can be easily transformed to a "one-step" compound inequality.

__

unfold

The equivalent compound inequality is ...

-4 < x +2 < 4

Subtract 2 to solve:

-6 < x < 2

_____

Additional comment

y = |x +2| means ...

`y=\begin{cases}-(x+2)&\text{for } (x+2) < 0\\(x+2)&\text{for }(x+2)\ge0\end{cases}`

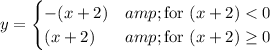
So, writing the equivalent pair of inequalities the "long way", we have ...

x +2 < 4 and (x +2) ≥ 0

and

-(x +2) < 4 and (x +2) ≤ 0

Multiplying the first part of the latter by -1, we can write it as a compound inequality.

(x +2) > -4 and (x +2) ≤ 0 ⇔ -4 < x +2 ≤ 0

Now, our original inequality is two compound inequalities:

-4 < x +2 ≤ 0 and 0 ≤ x +2 < 4

The union of their solution sets is ...

-4 < x+2 < 4