Question

Can anyone give me the following..

Excluded value(s):
LCD:
Equation after Multiplying by LCD:
Solution(s):

Answer 1

Solve for x over the real numbers:

2/(x - 6) + x/(x - 2) = 8/(x^2 - 8 x + 12)

Multiply both sides by x^2 - 8 x + 12:

2 (x - 2) + x (x - 6) = 8

Expand out terms of the left hand side:

x^2 - 4 x - 4 = 8

Add 4 to both sides:

x^2 - 4 x = 12

Add 4 to both sides:

x^2 - 4 x + 4 = 16

Write the left hand side as a square:

(x - 2)^2 = 16

Take the square root of both sides:

x - 2 = 4 or x - 2 = -4

Add 2 to both sides:

x = 6 or x - 2 = -4

Add 2 to both sides:

x = 6 or x = -2

2/(x - 6) + x/(x - 2) ⇒ 2/(-2 - 6) - 2/(-2 - 2) = 1/4

8/(x^2 - 8 x + 12) ⇒ 8/((-2)^2 - 8 (-2) + 12) = 1/4:

So this solution is correct

2/(x - 6) + x/(x - 2) ⇒ 2/(6 - 6) + 6/(6 - 2) = ∞^~

8/(x^2 - 8 x + 12) ⇒ 8/(6^2 - 8 6 + 12) = ∞^~:

So this solution is incorrect

The solution is:

Answer: |

| x = -2

Answer 2

• x = 2, 6 . . . excluded values
• (x^2 -8x +12) = (x -6)(x -2) . . . least common denominator
• (x^2 -4x -4)/(x^2 -8x +12) = 8/(x^2 -8x +12) . . . multiplied by LCD
• x = -2 . . . solution

Explanation:

A) Excluded Solutions

Values of x that must be excluded from the solution set are values that make any of the denominators be zero. Division by zero is undefined, so the equation is "undefined" for those values: they are not part of the domain of the equation.

Here, denominators are (x -6), (x -2), and (x^2 -8x +12). We can see immediately that x=6 will make the first of these be zero. x=2 will make the second of these be zero. When we factor the quadratic, we find it is ...

(x^2 -8x +12) = (x -2)(x -6)

This has the same zeros as the denominators we have already considered.

Excluded solutions: x = 2, 6

__

B) Least Common Denominator

As with numerical denominators, the "least common denominator" (LCD) is the product of the unique denominator factors, each raised to the highest power it has anywhere.

Here, the unique denominator factors are (x-6) and (x-2), so the LCD is ...

LCD = (x -6)(x -2) = (x^2 -8x +12)

__

C) Multiplied Equation

When we multiply the equation by the LCD, we get ...

`(2\cdot(x-6)(x-2))/(x-6)+(x\cdot(x-6)(x-2))/(x-2)=(8\cdot(x^2-8x+12))/(x^2-8x+12)\\\\2(x-2)+x(x-6)=8\qquad\text{cancel common factors}\\\\\boxed{x^2-4x-4=8}`

__

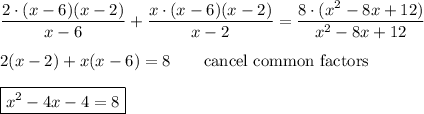
D) Solution

The equation resulting from multiplying by the LCD can be put in standard form and solved an any of the usual ways. Here, we find it convenient to factor it.

x^2 -4x -12 = 0 . . . . . . . subtract 8

(x -6)(x +2) = 0 . . . . . . . factor

Solutions to this equation are ...

x = 6, x = -2

We note that x = 6 must be excluded (see Part A), so the only solution is ...

x = -2

__

Alternate Solution

Extraneous solutions, such as x=6, can often be avoided by writing the original equation in the form f(x) = 0. Here, subtracting the right side gives a reduced equation with no extraneous solutions.

`(2)/(x-6)\cdot\left((x-2)/(x-2)\right)+(x)/(x-2)\cdot\left((x-6)/(x-6)\right)-(8)/((x-6)(x-2))=0\\\\(x^2-4x-12)/((x-6)(x-2))=0\qquad\text{simplify}\\\\((x-6)(x+2))/((x-6)(x-2))=0\qquad\text{factor}\\\\(x+2)/(x-2)=0\qquad\text{cancel common factors}\\\\\boxed{x=-2}\qquad\text{the solution makes the numerator zero}`

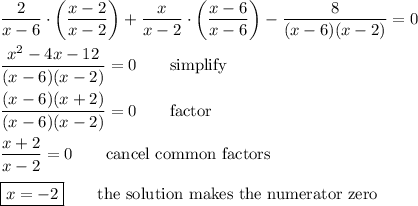
Note that this process allows the factor giving rise to the extraneous solution to be canceled. This simplifies the problem so we're solving a linear equation, x+2=0, rather than a quadratic.