Question

Find the slope of the line shown on the graph.

I need help with the three graph questions

Answer 1

Please check the explanation.

Explanation:

Graph from 1)

Given the points on graph 1

• (1, -2)

• (-3, 1)

Finding the slope between two points

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`\left(x_1,\:y_1\right)=\left(1,\:-2\right),\:\left(x_2,\:y_2\right)=\left(-3,\:1\right)`

`m=(1-\left(-2\right))/(-3-1)`

`m=-(3)/(4)`

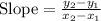
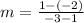
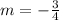
Graph from 2)

Given the points on graph 2

• (1, 4)

• (-3, -4)

Finding the slope between two points

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`\left(x_1,\:y_1\right)=\left(1,\:4\right),\:\left(x_2,\:y_2\right)=\left(-3,\:-4\right)`

`m=(-4-4)/(-3-1)`

`m=2`

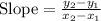
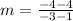
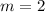
Graph from 3)

From figure 3, it is clear that the line is vertical.

Thus, the slope of a vertical line is undefined.