Answer:
The lines are parallel.
Explanation:
Given the lines
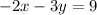

Writing the equations in the slope-intercept form

where m is the slope and b is the y-intercept
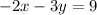
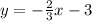
Thus, the slope = m₁ = -2/3
also

Thus, the slope = m₂ = -2/3
- We know that parallel lines have equal slopes
As
m₁ = m₂
Thus, the lines are parallel.