Question

Calculate the minimum frequency of ultrasound (in Hz) that will allow you to see details as small as 0.193 mm in human tissue. (Assume the speed of sound through human tissue is 1540 m/s.) What is the effective depth to which this sound is effective as a diagnostic probe?

Answer 1

f = 7.97 x 10⁶ Hz = 7.97 MHz

Step-by-step explanation:

The speed of a wave is given by the following formula:

`v = f\lambda`

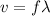
where,

v = speed of the ultrasound wave through human tissue = 1540 m/s

f = frequency of ultrasound wave required = ?

λ = wavelength of ultrasound waves = smallest detail required = 0.193 mm

λ = 0.193 mm = 1.93 x 10⁻⁴ m

Therefore,

`1540\ m/s = f(1.93\ x\ 10^(-4)\ m)\\f = (1540\ m/s)/(1.93\ x\ 10^(-4)\ m)`

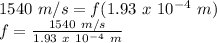
f = 7.97 x 10⁶ Hz = 7.97 MHz