Question

Hardy has three times as many tennis balls as kerns. They have 940 tennis balls in all. How many tennis balls does Hardy have than kerns?

Answer 1

Hardy has 470 more tennis balls than Kerns.

Explanation:

Given that:

Total number of tennis balls = 940

Let,

x represents the number of tennis balls Hardy has.

y represents the number of tennis balls Kerns has.

According to given statement,

x+y=940 Eqn 1

x = 3y Eqn 2

Putting x = 3y in Eqn 1

3y+y=940

4y=940

Dividing both sides by 4

`(4y)/(4)=(940)/(4)\\y=235`

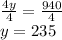
Putting y=235 in Eqn 2

x = 3(235)

x = 705

Difference = Hardy's tennis balls - Kerns' tennis balls

Difference = 705 - 235 = 470

Hence,

Hardy has 470 more tennis balls than Kerns.