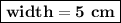Question

The area of a rectangle is 20 square meters. The length of the rectangle is (x-3) meters and the

width of the rectangle is (x - 2) meters. Find the length and the width of the rectangle.



With steps and explanation please.

Answer 1

length = 4 cm

Width = 5 cm

Explanation:

Area of rectangle:

Area of rectangle = length * width

length = (x - 3) meters

Width = (x - 2) meter

Area = 20 square meters

(x - 3)*(x - 2) = 20

Use FOIL method.

x*x + (x)(-2) + (-3)*x + (-3)*(-2) = 20

x² -2x - 3x + 6 =20

Combine like terms

x² - 5x + 6 = 20

Now subtract 20 from both side

x² - 5x + 6 - 20 = 0

x² - 5x - 14 = 0

Factorize the equation and find the roots

Sum = -5

Product = -14

Factors = (-7) , 2

When we add (-7) + 2 = -5 and when we multiply (-7)*2 = -14

x² + 2x - 7x - 14 = 0

x(x + 2) -7(x + 2) = 0

(x + 2)(x - 7) = 0

x - 7 = 0 {Ignore (x + 2 = 0) as measurements wont't be in negative}

x = 7

length = x - 3

= 7 - 3

`\sf \boxed{\bf length = 4 \ cm}`

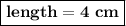
width = x - 2

= 7 - 2

`\sf \boxed{\bf width = 5 \ cm }`