Question

A stunt-rider on a motorcycle rides down a ramp and into a vertical loop-the-loop. If the diameter of the loop is 7.50 m, then the slowest speed the motorcycle can have at the top of the loop if it is to remain in contact with the loop is _________ km/h.

Answer 1

v = 6.06 m/s

Step-by-step explanation:

In order for the rider to pass the top of the loop without falling, his weight must be equal to the centripetal force:

`Centripetal Force = Weight \\(mv^2)/(r) = mg\\\\(v^2)/(r) = g\\\\v = √(gr)`

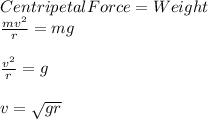
where,

v = minimum speed of motorcycle at top of the loop = ?

g = acceleration due to gravity = 9.8 m/s²

r = radius of the loop = diameter/2 = 7.5 m/2 = 3.75 m

Therefore, using these values in equation, we get:

`v = √((9.8\ m/s^2)(3.75\ m))`

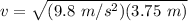
v = 6.06 m/s