Question

A person standing close to the edge on top of a 32-foot building throws a ball vertically upward. The quadratic function h(t)=−16t^2+124t+32 models the ball's height about the ground, h(t), in feet, t seconds after it was thrown. a) What is the maximum height of the ball? __feet b) How many seconds does it take until the ball hits the ground? ____ seconds

Answer 1

a) The maximum height of the ball is 272.25 feet

b) The balls take 8 seconds to hit the ground.

Explanation:

a) You know that the quadratic function h(t)=−16*t²+124*t+32 models the ball's height about the ground, h(t), in feet, t seconds after it was thrown.

Being a quadratic function of the form:

f (x) = a*x² + b*x + c

The vertex of a quadratic or parabola equation is the highest or lowest point on the graph corresponding to that function. When a> 0, the vertex of the parabola is at the bottom of it and indicates the minimum of the function. When a <0 the vertex is at the top and indicates the maximum of the function.

The value of x at the vertex is calculated by the expression:

`x=(-b)/(2*a)`

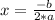
The value of y at the vertex is calculated by substituting the value of x at the vertex into the quadratic function.

In this case, being a=-16, b=124 and c=32 you get that the maximum time is:

`x=(-124)/(2*(-16))=3.875`

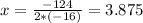
Then a maximum height of:

h(3.875)=−16*(3.875)²+124*3.875+32

h(3.875)= 272.25

The maximum height of the ball is 272.25 feet

b) When the ball hits the ground the height is zero. So h (t) = 0:

0=−16*t²+124*t+32

Applying the resolvent:

`\frac{-b+-\sqrt{b^(2) -4*a*c} }{2*a} =\frac{-124+-\sqrt{124^(2) -4*(-16)*32} }{2*(-16)}`

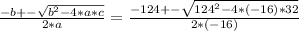
you get:

`\frac{-124+\sqrt{124^(2) -4*(-16)*32} }{2*(-16)} =-0.25`

`\frac{-124-\sqrt{124^(2) -4*(-16)*32} }{2*(-16)} =8`

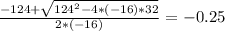
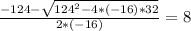
Since the time cannot be negative, the balls take 8 seconds to hit the ground.