QUESTION:
Simplify each expression
ANSWER:
1.)
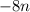
2.)
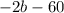
3.)
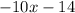
4.) for number 4 study my step-by-step explanation so you can answer that
STEP-BY-STEP EXPLANATION:
1.) First, If the term doesn't have a coefficients, it is considered that the coefficients is 1
WHY?
Learn why:
Why is it considered that the coefficient is 1?
Remember that any term multiplied by
 remains the same :
remains the same :

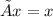
Step 1:
The equality can be read in the other way as a well, so any term can be written as a product of
 and itself:
and itself:
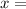


Step 2:
Usually, we don't need to write multiplacation sign between the coefficient and variable, so the simple form is:

This is why we can write the term without the coefficient as a term with coefficient

Now let's go back to solving as what i said if a term doesn't have a coefficient, it is considered that the coefficient is 1


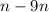
Second, Collect like terms by subtracting their coefficients

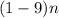
Third, Calculate the difference
how?
Keep the sign of the number with the larger absolute value and subtract the smaller absolute value from larger
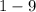
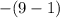
Subtract the numbers
- (
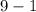 )n
)n
-
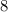 n
n
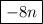
2.) First, Distribute - 6 through the parentheses
how?
Multiply each term in the parentheses by - 6
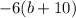
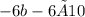
Multiply the numbers
-
 -
-

-
 -
-

Second, Collect like term
how?
Collect like terms by calculating the sum or difference of their coefficient
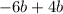

Calculate the sum
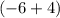 b
b
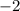 b
b
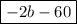
3.) First, Distribute 2 through parentheses
how?
Multiply each term in the parentheses by 2
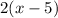
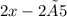
Multiply the numbers
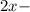
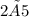
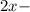
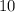
Second, Distribute - 4 through the parentheses
how?
Multiply each term in the parentheses by - 4
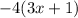
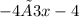
Calculate the product
-
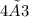 x - 4
x - 4
-
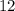 x - 4
x - 4
Third, Collect like terms
how?
Collect like terms by subtracting their coefficient

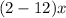
Calculate the difference
 x
x
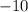 x
x
Fourth, Calculate the difference
how?
Factor out the negative sign from the expression
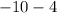

Add the numbers
- (
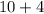 )
)
-
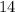
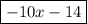
That's all I know sorry but I hope it helps :)