Question

If the measurment of the angles of a triangle are 3b,2b,and 4b then what is the smallest angle

Answer 1

Given:

The measurement of the angles of a triangle are 3b,2b,and 4b.

To find:

The smallest angle.

Solution:

According to the angle sum property, the sum of all angles of a triangle is 180 degrees.

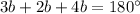`3b+2b+4b=180^(\circ)` [Angle sum property]

`9b=180^(\circ)`

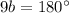
Divide both sides by 9.

`b=20^(\circ)`

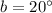
Now,

`3b=3(20^(\circ))=60^(\circ)`

`2b=2(20^(\circ))=40^(\circ)`

`3b=4(20^(\circ))=80^(\circ)`

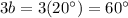
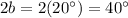
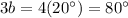
Therefore, the smallest angle is 40 degrees.